Matrix properties
number of rows	32,768
number of columns	32,768
nonzeros	98,300
# strongly connected comp.	1
explicit zero entries	0
nonzero pattern symmetry	symmetric
numeric value symmetry	symmetric
type	binary
structure	symmetric
Cholesky candidate?	no
positive definite?	no

author	R. Diekmann, R. Preis
editor	R. Diekmann, R. Preis
date	1998
kind	undirected graph sequence
2D/3D problem?	no

Additional fields	size and type
G	cell 13-by-1
Gname	cell 13-by-1

Notes:

AG-Monien Graph Collection, Ralf Diekmann and Robert Preis                     
http://www2.cs.uni-paderborn.de/fachbereich/AG/monien/RESEARCH/PART/graphs.html
                                                                               
A collection of test graphs from various sources.  Many of the graphs          
include XY or XYZ coordinates.  This set also includes some graphs from        
the Harwell-Boeing collection, the NASA matrices, and some random matrices     
which are not included here in the AG-Monien/ group of the UF Collection.      
In addition, two graphs already appear in other groups:                        
                                                                               
   AG-Monien/big : same as Nasa/barth5, Pothen/barth5 (not included here)      
   AG-Monien/cage_3_11 : same as Pajek/GD98_c (included here)                  
                                                                               
The AG-Monien/GRID subset is not included.  It contains square grids that      
are already well-represented in the UF Collection.                             
                                                                               
Six of the problem sets are included as sequences, each sequence being         
a single problem instance in the UF Collection:                                
                                                                               
   bfly:  10 butterfly graphs 3..12                                            
   cage:  45 cage graphs 3..12                                                 
   cca:   10 cube-connected cycle graphs, no wrap                              
   ccc:   10 cube-connected cycle graphs, with wrap                            
   debr:  18 De Bruijn graphs                                                  
   se:    13 shuffle-exchange graphs                                           
                                                                               
Problem.aux.G{:} are the graphs in these 6 sequences.  Problem.aux.Gname{:}    
are the original names of each graph, and Problemm.aux.Gcoord{:} are the       
xy or xyz coordinates of each node, if present.                                
                                                                               
Graphs in the se sequence:                                                     
                                                                               
     1 : SE3          :       8 nodes      10 edges      20 nonzeros           
     2 : SE4          :      16 nodes      21 edges      42 nonzeros           
     3 : SE5          :      32 nodes      46 edges      92 nonzeros           
     4 : SE6          :      64 nodes      93 edges     186 nonzeros           
     5 : SE7          :     128 nodes     190 edges     380 nonzeros           
     6 : SE8          :     256 nodes     381 edges     762 nonzeros           
     7 : SE9          :     512 nodes     766 edges    1532 nonzeros           
     8 : SE10         :    1024 nodes    1533 edges    3066 nonzeros           
     9 : SE11         :    2048 nodes    3070 edges    6140 nonzeros           
    10 : SE12         :    4096 nodes    6141 edges   12282 nonzeros           
    11 : SE13         :    8192 nodes   12286 edges   24572 nonzeros           
    12 : SE14         :   16384 nodes   24573 edges   49146 nonzeros           
    13 : SE15         :   32768 nodes   49150 edges   98300 nonzeros           
                                                                               
The primary graph (Problem.A) in this sequence is the last graph               
in the sequence.                                                               

SVD-based statistics:
norm(A)	2.99998
min(svd(A))	7.96067e-19
cond(A)	3.7685e+18
rank(A)	32,311
null space dimension	457
full numerical rank?	no
singular value gap	2.42338e+12

singular values (MAT file):	click here
SVD method used:	s = svd (full (A))
status:	ok

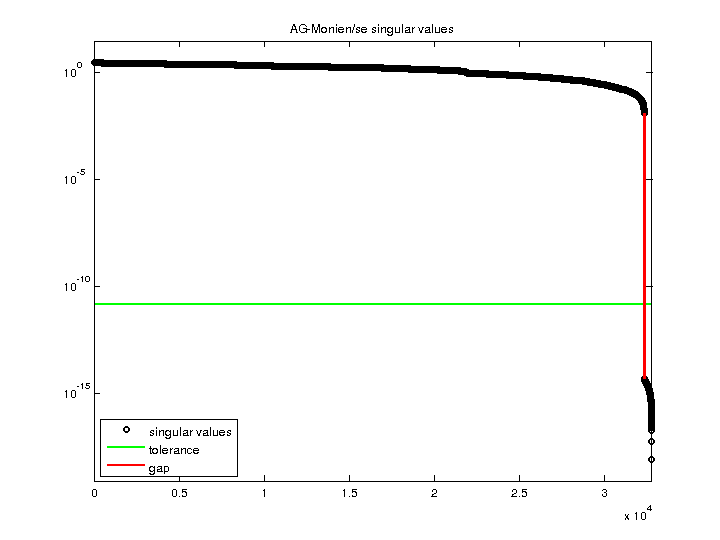
For a description of the statistics displayed above, click here.

Maintained by Tim Davis, last updated 12-Mar-2014.
Matrix pictures by cspy, a MATLAB function in the CSparse package.
Matrix graphs by Yifan Hu, AT&T Labs Visualization Group.